A New Method for Prediction of Dental Arch Perimeter

Introduction

Dental arch perimeter (or length) is considered as one of the most important dental arch parameters for the diagnosis of orthodontic cases.¹

Daskalogiannakis² defines it as the space available in the dental arch for the alignment of the teeth. Crowding of teeth is considered as a major problem that affects the dental arch; it can be resolved by careful analysis of the space available (arch perimeter) and space required (teeth width) and an appropriate method for space creation and/or preservation.^3,4 It is equally important to increase awareness about dental health by minimizing the incidence of caries, which lead to the loss of dental material and could affect the dental arch perimeter.⁵

Several methods for calculating the dental arch perimeter have been adopted by various authors. One of these methods involves direct measurement of these parameters by extending a brass wire^6,7 or steel wire⁸ along the distances that need to be measured and then straightening and measuring the length of the wire. This method is affected by dental irregularities such as crowding, rotation, and/or displacement of the teeth. Therefore, it is not so dependable, especially for determining the line of occlusion, on account of differences in geometric form and arch length, and it requires considerable judgment about the proper arch form.^9–11

Alternatively, Musich and Ackerman¹² used the catenometer for direct measurement of the dental arch perimeter. Their method is generally considered as being rapid and reliable for determining the mandibular arch perimeter. Another method entails dividing the dental arch into four,^13–18 five,¹⁹ or six segments,²⁰ and the sum of the measurements for these segments is considered to represent the dental arch perimeter. The segmented arch technique is regarded as a very easy, precise, and satisfactory method for measuring the dental arch perimeter.

Yet another method is the electronic X-Y plotter method, in which the X-Y coordinates of select reference points are determined, and the digital co-ordinates are analyzed with a linked computer program.²¹ However, although this procedure is quick, it requires a suitable computer program that must be set up with information in order to avoid tedious manual measurements. Thus, these methods mentioned above are generally in use, but they have certain limitations that need to be addressed.

The type of method that is the focus of this study is a mathematical one that uses multiple regression analysis to develop an equation for estimating arch perimeter, as described by Sanin et al,²² Paulino et al²³ and Al-Khatieeb et al.²⁴ This method involves analysis of the relationship between arch lengths, widths, and perimeters.

Sanin et al²² found that dental arch width and length had a direct, strong relationship with the dental arch perimeter; thus, they developed the following regression equation to predict dental arch perimeter: Dental arch perimeter= (dental arch width × 0.504) + (dental arch length × 1.525) + 14.856. This equation is believed to be accurate. In 2008, Paulino et al²³ proposed a regression equation that utilized inter-canine width to predict arch perimeter (referred to as arch length in their article): Arch length (perimeter) = (1.36 × inter-canine width) + 29.39. In 2014, Al-Khatieeb et al²⁴ tried to predict arch perimeter based on dental arch width at the level of each tooth: 35.07+ 2.59(R1-L1) + 0.18(R2-L2) + 0.03(R3-L3) + 0.10(R4-L4) + 0.003(R5-L5) + 0.10(R6-L6) for the maxillary arch, and 15.66 + 4.19 (R1-L1) + 0.21(R2-L2) + 0.05(R3-L3) + 0.47 (R4-L4) + 0.23(R5-L5) + 0.10 (R6-L6) for the mandibular arch.

Ricketts et al²⁵ found that the arch length increased by 1 and 0.25 mm for each 1 mm increase in the inter-canine and inter-molar distances, respectively, but their method of assessment was not published. Alternatively, a parabolic curve equation^26,27 and Ramanujan’s equation for measurement of the ellipse^28,29 have also been proposed.

The aim of the present study is to develop new regression equations that utilize dental arch width and length to predict the dental arch perimeter.

Materials and Methods

Samples

The study sample includes 67 pairs of models that belong to Iraqi Arab subjects who received dental treatment at the dental clinics at Al-Rafidain University College, Baghdad, Iraq. The participants had a complete set of permanent dentition and class I dental relationship with less than 3mm crowding or spacing, with no history of abnormal oral habits, trauma, craniofacial anomalies, or gingival and periodontal problems.

Methods

This study was approved by the ethical and scientific committees of the College of Dentistry of Baghdad and Al-Rafidain University College and carried out in accordance with the principles of the Declaration of Helsinki. Written informed consent was obtained by the subjects for participation in this study.

intra- and extra-oral clinical examinations were performed to determine whether the participants met the inclusion criteria, then dental impressions of the maxillary and mandibular arches were taken using alginate impression materials (Chromatic alginate Tropicalgin; Zhermack, Italy). The impressions obtained were washed and disinfected with 1:10 sodium hypochlorite,³⁰ and then used to create dental stone casts (Elite Rock, Sandy brown; Zhermack, Italy).

The width, length, and perimeter of the dental arches were measured using a digital vernier gauge (Mitutoyo, Japan), with 0.01mm accuracy. The width was measured as the linear distance at the level of the cusp tip of the canines, buccal cusps of the first premolars, and mesiobuccal cusps of the first molars.^31,32

The length was determined as the vertical distance between the central point of the two central incisors and the line joining the mesiobuccal cusp tips of the first molars.¹⁵

Dental arch perimeter was measured as the sum of five segments: from the mesial point of the first molars to the distal point of the canines, from the distal point of the canines to the distal point of the central incisors on both sides, and from the distal point of the right central incisors to the distal point of the left central incisors.^19,33

Statistical Analysis

The data were subjected to statistical analyses using the SPSS software (version 25; IBM Co., New York, USA). The means, standard deviations, and minimum and maximum values were determined for each variable. Pearson’s correlation coefficient test was used to assess the relationship of the dental arch perimeter with the dental arch width and length.

Step-wise linear regression analysis was used to determine the predictor(s) of dental arch perimeters. After the regression equations were applied, the actual and predicted arch perimeters were compared using a paired sample t-test. The probability value (P-value) was set at 5%.

Results

The descriptive statistics of the measured parameters are presented in Table 1. The relation between the dental arch perimeter and other variables for both arches is presented in Table 2.

Table 1 Descriptive Statistics of the Parameters Measured (mm)

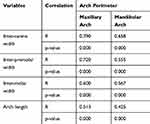Table 2 Correlation Between Dental Arch Perimeter and Other Parameters in Both Arches

The inter-canine and inter-first premolar width showed a direct, strong, and highly significant correlation with the maxillary arch perimeter. Inter-molar width and arch length showed a direct, moderate, and highly significant correlation with the maxillary arch perimeter (P<0.001). On the other hand, the dental arch perimeter had a direct, moderate, and highly significant correlation with other parameters in the mandibular arch (P<0.001).

After the data were subjected to step-wise regression analysis, two regression equations were developed—one each for the maxillary and mandibular arches (Table 3). For the maxillary arch, inter-canine width and arch length showed a strong direct correlation with arch perimeter (r= 0.823), and were therefore identified as predictors of arch perimeter. In Pearson’s correlation analysis, any variation in one variable can be explained by variation in another variable. Accordingly, in the present analysis, inter-canine width and arch length explained 67.7% of the variation in dental arch perimeter (r²=0.677).

Table 3 Regression Equations for Predicting Dental Arch Perimeters

With regard to the mandibular arch, inter-molar width, inter-canine width, and arch length showed a strong direct correlation with arch perimeter (r= 0.742). These three variables explained 55.1% of the variation in dental arch perimeter (r²= 0.551).

A paired sample t-test was used to compare the actual and predicted arch perimeters (Table 4), and the results indicated that there was no significant difference in the predicted and actual (P>0.05).

Table 4 Comparison of Actual and Predicted Arch Perimeters

Discussion

To reach definitive diagnosis for every orthodontic case, information from case history, panoramic and lateral cephalometric X-rays in addition to study models must be gathered and interpreted carefully.³⁴ In the last years, cone beam computed tomography offered many advantages with regard of measuring the dental arches’ widths lengths and perimeters³¹ besides its advantage in evaluating impacted teeth and bone density and dental implant position determination. This type of image can not be used for any patient without justification because of radiation hazards.

Study models offer many advantages like the determination of Bolton’s ratios, space available and required, arch widths, lengths and perimeters with the aid of vernier or analyzing softwares like OrthoCad.³⁵ It has been found that digital models generated from CBCT scan are not perfect to be used in model analysis like the plaster or some digital models.³⁶

In the present study, dental arch width at different levels, as well as dental arch length, were found to be correlated with the dental arch perimeter for both the mandibular and maxillary arch. Importantly, the correlation was direct and highly significant for all measurements.

After the data were analyzed with step-wise regression analysis, only inter-canine width and arch length in the maxillary arch, and inter-canine width, inter-molar width, and arch length in the mandibular arch still presented with a strong correlation with the dental arch perimeters, while the other parameters were excluded.

According to the findings of step-wise regression analysis, regression equations were developed for the maxillary and mandibular arch. The obtained predicted dental arch perimeter values were compared with the actual ones using paired sample t-test, and the results revealed that there was no significant difference.

The present study is considered as the fourth study, after that of Sanin et al,²² to predict dental arch perimeter from arch width and length. It must be noted that Paulino et al²³ and Al-Khatieeb et al²⁴ used only dental arch width to predict arch perimeter. The equations of the present study differ from previously reported ones because of differences in the method of measurement used and criteria applied for sample selection. Additionally, while Paulino et al²³ developed one equation for both arches, we have developed separate equations for the mandibular and maxillary arches that may differ in their dimensions.

Further studies are required on methods for predicting dental arch perimeter in the case of crowded, spaced dentition and class II and III malocclusions.

Conclusions

Dental arch length and width were employed to develop new regression equations to predict dental arch perimeters for the maxillary and mandibular arches, and the predicted perimeters did not differ significantly (P>0.05) from the measured ones. Thus, these equations would be highly useful in diagnosis and treatment planning.